Ascolta

 

Research projects

 

Dynamic analysis and monitoring

The analysis of the dynamic behavior of structures is very important since the most severe natural events (earthquake and wind) are phenomena which induce dynamic loads on structures that can be characterized only through adequate monitoring project. From the results of these investigations essential information can be drawn for the structural engineer who, through mathematical models and more and more advanced computational techniques, can provide data on the conditions of the structure, and the possible actions to be taken. In this context it emerges the importance of the structural dynamic analysis in particular in the field of protection of historic monuments and cultural and architectural heritage of which the Sicilian region is particularly rich. It is also important to consider the structural monitoring with non-destructive techniques thanks to which it is possible to detect the presence of micro or macro fractures in solids and monitoring their propagation through the use of non-destructive techniques such as the method of Acoustic Emission (passive method) and Ultrasonic method (active method) .

Multiscale analyses with application to periodic structures

This research activity is devoted to develop multi-scale systems for the computational analysis of structures constituted by heterogeneous materials where it is possible to identify a periodic representative volume element. The multi-scale analyses contribute to a reduction of computational costs thanks to a separation of the scale of interest. It is possible to distinguish between a macroscopic scale (with dimensions of the structure, that is considered as an homogeneous continuum) and a mesoscopic scale (with dimensions of constituents, that are individually modelled).

Tissue biomechanics and mec-biology

In this field the research activity has been devoted to the determination of physical-mathematical models able to describe the behavior of biological tissues and cell aggregates present in the parenchyma of more complex organs. In this regard predictive models of the hereditary mechanical behavior of collagen tissues, more or less mineralized, and muscle tissues have been developed by the use of fractional differential calculus. The study of cell aggregates, which are involved in the parenchyma of more complex organs, has been carried out by the use of fractal geometry for the determination of the mechanical characteristics of the aggregate. Dynamic models able to predict the behavior of cellular and nuclear hereditary lipid membranes are also under study, experimental evidences show the presence of marked hereditariness of the membrane response at orthogonal plane of the membrane. In the mec-biology field a predictive model of mediated endocytosis based on fractional differential calculus for the description of the motion of membranal receptors towards the corresponding ligands has been developed.

Stochastic differential calculus

In structural engineering many of the dynamic actions on structures are random processes also known as stochastic; this category includes earthquakes, gusts of wind and waves motion. The structural response to these actions is also a random process. It must therefore be characterized "probabilistically" through the tools of stochastic differential calculus .

Despite the large number of cases in which it is possible to characterize the external loadings as Gaussian normal processes, sometimes in order to be more accurate in modeling physical reality, not Gaussian not normal processes have to be considered. Within the study of linear and non-linear systems under normal white noise and/or non-normal processes, novel methods are proposed for the solution of Fokker–Planck or Kolmogorov- Feller differential equation to describe the system in terms of displacement probability density function.

Analysis of continuous beams with viscoelastic behavior by fractional calculus

In recent years, modern production techniques have allowed to obtain structural materials with innovative mechanical characteristics that are significantly higher than the traditional ones commonly used in the structures, i.e. the polymeric materials, the nano-composites, the bio-inspired tissues, the sandwich composites, the multiphase materials etc.. A particular feature of these new materials, which distinguishes them from traditional materials, is the fact of not having a perfectly elastic behavior. In fact, these materials show marked time-deferred phenomena due to their viscoelastic nature. To adequately characterize the viscoelastic behavior it should be considered constitutive laws in which the state of tension is related to the derivative of fractional order a of deformation respect to time, simulating it as an interpolating behavior between the two boundary cases: elastic and viscous, with abetween 0 and 1; the perfectly elastic case is obtained when the order of fractional derivative of the deformation is a=0 while the perfectly viscous one is given when a=1. The latter type of model, known as fractional viscoelastic model, simulating effectively the real mechanical behavior of materials, represents the innovative model that is the most appropriate to study the structural response of continuous systems.

Vibration control

The trend towards the use of materials with better mechanical strength characteristics, together with the use of the limit analysis method, leads to the creation of structures increasingly slender and deformable, for which the reduction of vibrations is certainly a major challenge. One of the main goals of researchers and designers in the context of structural engineering is therefore the study for the design of innovative devices that induce a reduction of structural vibrations due to dynamic loads such as wind or earthquake.

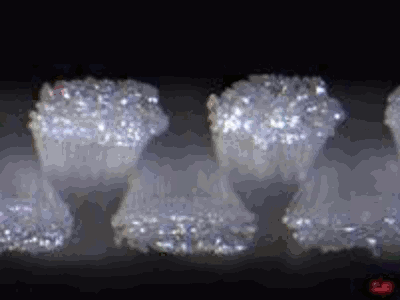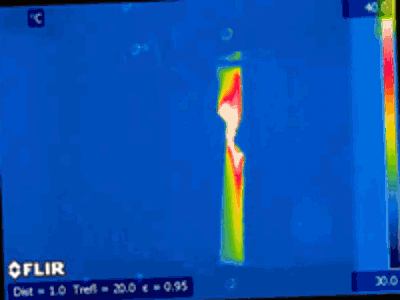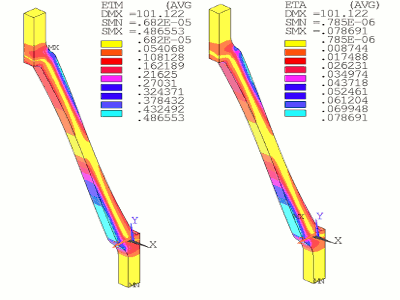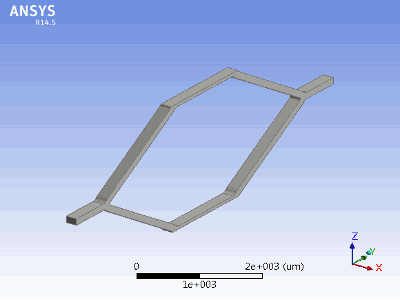
Computational mechanics

• Use of the Boundary Element Method in its symmetric formulation. It has been written also a computer code, called Karnak.sGbem that is in continuous developing, in order to perform numerical simulations in various fields of mechanics:

• • Approach to sub-structures;
  • Evaluation of the energy in a subspace;
  • Fracture of brittle materials;
  • Cohesive fracture mechanics in quasi-brittle materials;
  • Limit and shakedown analysis;
  • Incremental elastic plastic analysis associated with the problem of contact-detachment.
    

• Shear and torsion stress analysis of solid with the LEM method (line elementless method)

The problem of solid stressed by shear and torsion is solved through the computation of line integrals, with no need to discretize the domain or the boundary of the section. The method is "robust" in the sense that returns the exact solution for those sections in which the exact solution exists.

Mechanics of coupled problems: Thermoelasticity and poroelasticity

The research group of Palermo developed new theories for thermal energy and mass transport corresponding, at the macroscale to transport equations involving fractional differential operators. The physical context corresponding to fractional-order operators have been obtained in terms of thermal energy transport and fluid flow across porous media with appropriate spatial grading of the mechanical and geometrical features. A similar result has been obtained considering a thermal energy flux and/or the mass transport associated to the flow of a Newtonian fluida cross a fractal porous media. The net outgoing flux is ruled, in such cases, by fractional-order operators with differentiation order related to the fractal dimension of the set. The fractional-order transport equation have then been applied to multifield physical problems in thermoelasticity and poroelasticity.

Mesomodeling of structures constituted by heterogeneous materials

Developing of original interface or interphase models to carry numerical finite element analyses with applications to adhesive\cohesive joints of quasi-brittle materials. Particularly important are those analyses dedicated to structures constituted by heterogeneous materials such as masonry or composites.

Nanomechanics

The research activity in this field involves the mechanical model of nanotubes, nanostructures, nanobeams and bio-inspired materials in terms of hierarchic materials to evaluate their elastic properties as well as their strength. The tools used to study such problem are the non-local, displacement based elasticity theory recently proposed by the Palermo research group that allows to describe the long-range intermolecular forces at the nanometric scales, in terms of integro-differential equations. The studies conducted at DICAM facility regard continualization theories, statics, dynamics, wave propagations, elastic stability, damped vibrations. Some other current researches involves nanocomposites homogenization in presence of hereditary inclusions.

Structural optimization

Structural optimization is a relatively new research area that in the last decades has been subjected to wide advancements both in terms of theoretical formulations that of computational techniques. In addition, in engineering practice is widely used and is a safe reference. The produced formulations and the related numerical approaches allow to obtain optimal designs of simple and complex structures subject to static or dynamic loads or even to appropriate combinations of them, considering the elastic and the elastoplastic behavior. Particular attention has been devoted to the case of seismic loads and recent studies allow to take into account their random nature. It has been treated also the current case of base isolated structures.

Hereditariness in mechanics of materials

The research activity in this field has been focused on the physical model corresponding to power-law creeep/relaxation showed by almost every real material. In this context the research group of Palermo introduced a mechanical model that is exactly corresponding to power-law relaxation and creep functions and that is sensitive to elasto-viscous and visco-elastic materials depending upon the prevalence of the elastic or the viscous phase of the material. Such a distinction corresponds to a differentiation order lower or higher of 0.5, respectively. The phase separation has also allowed to evaluate, exactly, the free energy stored in the material for any strain process that coalesces with the Stavermann-Schwarz free energy obtained, directly, from creep and relaxation measures. In this setting the free energy function in presence of large configurational changes has also been obtained by means of the logarithmic strain.

Research projects

• PRIN 2009: A multi-scale approach for the analysis of decohesion processes and fracture propagation”. Scientific Coordinator: Prof. Alberto Corigliano. Scientific Referent for the Research Unit of Palermo: Prof. Guido Borino.
• PRIN 2010-11: Models and algorithms for the nonlinear analysis of structures and the validation of performance-based design rules. Scientific Coordinator:Prof. Raffaele Casciaro. Scientific Referent for the Research Unit of Palermo: Prof. Teotista Panzeca.
• PRIN 2008: A physical approach to the non-local viscoelasticity and phase change: Applications to the fracture mechanics, Scientific Coordinator: CARPINTERI Alberto, Scientific Referent for the Research Unit of Palermo: Mario Di Paola.
• PRIN 2013- Dynamics, stability and control of flexible structures, Scientific Coordinator: Prof. AngeloLuongo. Scientific Referent for the Research Unit of Palermo: Prof. Mario Di Paola.
• Progetto SLIM (POR 4.1.1) 2013:P rof. Mario Di Paola.